

There are many factors involved in maximizing performance. A lot depends on what you call performance. The term "performance" can have a number of meanings, depending on the type of vehicle and the results you’re looking for. Performance may be how fast it goes around a corner, how well it brakes, or its acceleration in a straight line.
It’s usually safe to say "bigger equals better, faster and more money at the winner’s circle." One factor in faster is getting the horsepower to the ground. Most people, after creating their biggest, baddest, most powerful (and usually most expensive) engine, will focus on the transmission and differential, hoping to find just the right combination of ratios to make their way to the finish line first.
Many people look to some of the older transmissions to fit their needs. Indeed, many still use the Powerglide for certain racing applications, especially drag racing. This brings up the often asked question: "What is the ratio of this transmission?" This edition of "Shop Talk" is dedicated to the arithmetic of figuring the ratios in an automatic transmission.
Planetary geartrains are way over complicated. In essence, the ratio is the output gear divided by the input gear—just like with a differential. For example, if a differential has a ring gear with 122 teeth, and a pinion with 48 teeth, the ratio would be 122 ÷ 48, which equals 2.54, or 2.54:1.
The planetary gearset is no different. The basic planetary gearset consists of a sun gear, ring gear, and planet (figure 1). The question is, which gear is the output, and which is the input? That depends on which components are turning, and which are being held: The idler gears of the planet are never considered in the calculation—only the sun gear and ring gear figure into the equation. Another thing to remember is that a single planetary gearset can offer four functions: reduction, overdrive, direct drive, and reverse.
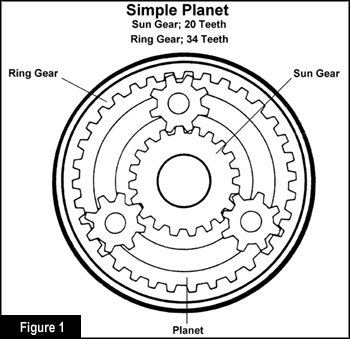
|
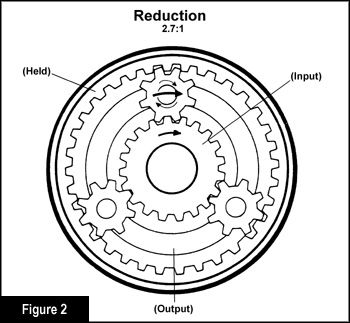
Reduction Gear reduction is always accomplished by using the planet as the output, and either the ring gear or sun gear as the input. But remember, even though the planet is the output device it isn’t considered in the calculations. The third component (either sun gear or ring gear) is held stationary. For the first example we’ll use the sun gear as the input. So here’s that question again: What do we use for the output? It’s the tooth count of the ring gear plus the sun gear. Why? That’s because, while the sun gear is turning, the planet is rotating, and also walking between both the sun gear and ring gear ... so you count both of them as the output. This rule is in effect whenever the planet rotates independently of the sun gear and ring gear. If the ring gear were the input and the planet the output, the calculation would be slightly different: The ring gear plus the sun gear (output), divided by the ring gear (input). For the following examples, let’s use a ring gear with 34 teeth and a sun gear with 20 teeth. If the ring gear was held stationary and the sun was the input, the formula would be: (34 + 20) ÷ 20 = 2.7:1 (figure 2). |
|
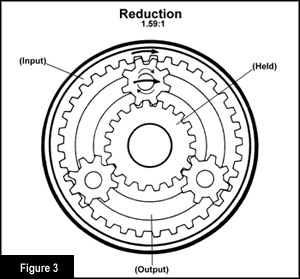
Now let’s look at what happens when the ring gear is used for the input and the sun gear is held stationary. Now the formula is slightly different: (34 + 20) ÷ 34 = 1.59:1... much different (figure 3). But notice that the same count (34 + 20) is used for the dividend (the number being divided) in both equations.
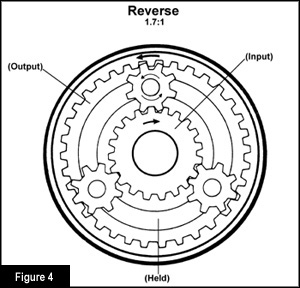
Overdrive
Let’s look at a couple of examples: If the ring gear was held stationary and the sun gear was the output? The input is the sum of the ring gear and sun gear. So the formula would be 20 ÷ (34 + 20) = 0.37:1 (figure 4). Some overdrive, huh?
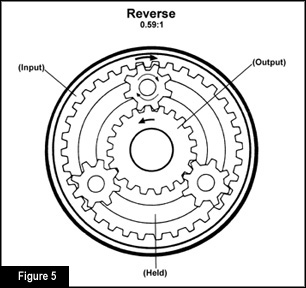
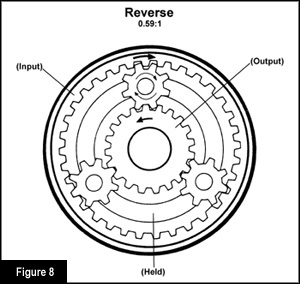
Most manufacturers use the ring gear as the output, so let’s try that. The formula for this is 34 ÷ (34 + 20) = 0.63:1 (figure 5). Now that’s more like it.
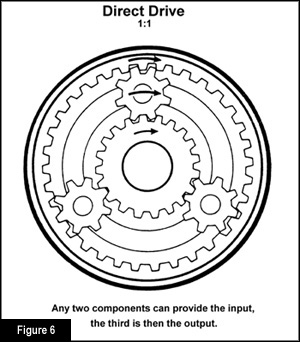
Direct Drive
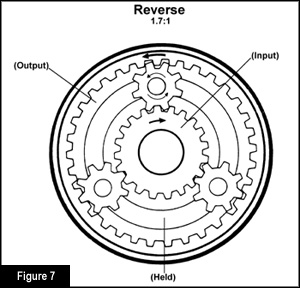
Reverse
Compound Gearsets
One of the earliest compound gearsets used in automatic transmissions is the Ravigneaux gearset (figure 9). The Ravigneaux gearset appears in the FMX, AOD and T-65, among others. It has compound gears with long and short pinions. It uses one ring gear and two sun gears. In most cases, the small sun gear provides forward input, and the large sun gear provides the reverse input, direct drive, second brake and overdrive brake.
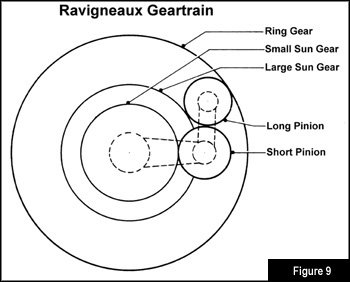
Use the same idea to calculate ratios: the input divided by the output. But once again, the trick is figuring out what is the input, and what is the output. For this example, let’s use an AOD. Its ring gear has 72 teeth. The small sun gear has 30 teeth and the large one has 36. Now remember, the pinions are simply idler gears, so they aren’t used in the calculation.
First gear is easy: It’s simply the ring gear divided by the small sun gear, 72 ÷ 30 = 2.4:1. But earlier we said that when the plant is held stationary, you get reverse. A closer look reveals that the long pinions of the planet provide for a double pinion, reversing the output. Kind of like reversing reverse.
Second gear is the trickiest because there are really two gearset functions going on at the same time. The first is strictly with the sun gears: The large sun gear is held stationary and the small sun gear is the input. What about the output? Because the planet is walking around both sun gears, they are both used as the output. So the calculation is: (30 + 36) ÷ 30 = 2.2:1. But we’re not finished yet. To make the next part easier to understand, get an AOD gearset, and turn it as it would turn for second gear—turning the small sun gear while holding the large sun gear stationary, but without the ring gear in place. Notice that this action is used simply to turn the plant. As far as the ring gear is concerned, the planet is now an input. Remember what we said before: When the planet provides the input, you get overdrive. So the second part of the formula is an overdrive ratio. The ring gear is the output; the input is the ring gear plus the large sun gear (because it is being held stationary). So the calculation is 72 ÷ (72 + 36) = 0.67:1. This two ratio is combined with the earlier ratio of 2.2:1. The result is 2.2 x 0.67 = 1.47:1. Essentially it is an overdriven first gear.
Third gear is the easiest: it’s 1:1. The entire geartrain is spinning as a single unit.
Overdrive is a little tricky, but we’ve already done it during the calculation for second. Think about it: The reverse drum is held stationary and the planet is the input. The only difference here is, in second, the input that turned the planet was a gear reduction of 2.2:1. In overdrive, the direct drum turns the planet at 1:1, so we only use the overdrive calculation, which gives us 0.67:1.
Reverse uses the large sun gear as the input and the ring gear as the output: The planet is held stationary. The formula for this is 72 ÷ 36 = 2:1
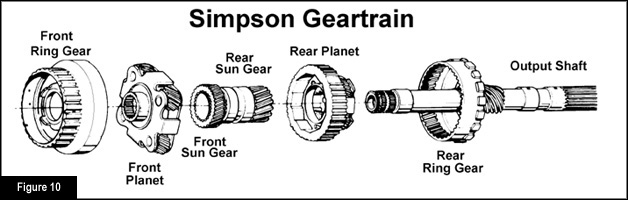
Another very common gearset is the Simpson gearset. The first transmission to use it was the Aluminum Torqueflite; the example in figure 10 is from a THM 200. The Simpson gearset uses two planets and a common sun gear. The ring gear for the front plant is the forward input and the rear ring gear splines to the output shaft.
Most Simpson geartrains use the same tooth count for the front and rear ring gears. And they have one long sun gear, so the tooth count for the sun gears are the same. For example, the 350, and the C6. The 400 is sort of a backward Simpson, but it, too, uses the same tooth count for both ring gears and both sun gears.
In this example though, let’s use a 350. The 350 ring gears each have 66 teeth. The sun gear has 34 teeth. For first gear, the torque goes through the front ring gear to the sun gear, then to the rear ring gear. Because all three gears are used, all three will count as the output. The formula for first gear is (66 + 66 + 34) ÷ 66 = 2.52:1.
Second gear is easy: Only the front planetary is used. The formula for this is the front ring gear plus front sun gear, divided by the front ring gear: (66 + 34) ÷ 66 = 1.52:1.
Notice the relationship between the formulas for first and second gears: The difference in the ratio of the first two formulas is "1." With the two formulas next to each other, it’s easy to see the difference (66 + 66 + 34) ÷ 66 and (66 + 34) ÷ 66. There is one more "66" in the first formula. So all you really need to do is figure out the second gear ratio, then add "1" to it for the first gear ratio. This will work on all Simpson gearsets that use the same number of teeth on both ring gears and both sun gears.
Once again third gear is 1-to-1: The entire geartrain is rotating as a set.
Now let’s look at reverse. Reverse is accomplished the same way as the Ravendeaux gearset. The rear planet is held stationary while the sun gear provides the input and the ring gear provides the output. So it’s simply the rear ring gear divided by the rear sun gear: 66 ÷ 34 = 1.94:1.
That one was pretty easy. But there are some Simpson gearsets that are a little more difficult: They’re the ones that use different front and rear sun gear and ring gear counts, such as the 200 and E4OD. Second and third gear use the same formula as the 350, but the formula for first is way different.
Let’s review the 350, then we’ll look at the difference: For the 350 (or any Simpson gearset with the same tooth count for both ring gears and both sun gears), the output is the sum of the rear ring gear, sun gear and the front ring gear.
But when the two sun gears are connected and have different tooth counts, they act almost like their own separate gearset. In other words, for each rotation of the sun gear, the front planet sees 42 teeth and the rear planet sees 30: This affects the entire formula. Because of this difference, we must find the ratio of this effect, and figure it into the rear ring gear’s tooth count.
So the rear ring gear’s effective tooth count is the rear ring gear divided by the rear sun gear, multiplied by the front sun gear 62 ÷ 30 x 42 = 86.79. This is the effective rear ring gear tooth count. Now continue with the formula: The effective rear ring gear, plus the front sun gear, plus the front ring gear, divided by the front ring gear, or (86.79 + 42 + 74) ÷ 74 = 2.74:1.
There are other types of gearsets used in different transmissions, and the methods used to arrive at their ratios are pretty simple. Just remember the rules for a single planet for figuring a gear reduction, overdrive or reverse. And remember, when a planet walks with a ring gear or sun gear, the sun gear and ring gear tooth counts are added together to make up either the input or output. Once you’ve got that, you’ll always be ready to handle the arithmetic of performance gearing.
![]()